Solving physical problems or examples in mathematics is completely impossible without knowledge of the derivative and methods for calculating it. The derivative is one of the most important concepts in mathematical analysis. We decided to devote today’s article to this fundamental topic. What is a derivative, what is its physical and geometric meaning, how to calculate the derivative of a function? All these questions can be combined into one: how to understand the derivative?
Geometric and physical meaning of derivative
Let there be a function f(x) , specified in a certain interval (a, b) . Points x and x0 belong to this interval. When x changes, the function itself changes. Changing the argument - the difference in its values x-x0 . This difference is written as delta x and is called argument increment. A change or increment of a function is the difference between the values of a function at two points. Definition of derivative:
The derivative of a function at a point is the limit of the ratio of the increment of the function at a given point to the increment of the argument when the latter tends to zero.
Otherwise it can be written like this:
What's the point of finding such a limit? And here's what it is:
the derivative of a function at a point is equal to the tangent of the angle between the OX axis and the tangent to the graph of the function at a given point.
Physical meaning of the derivative: the derivative of the path with respect to time is equal to the speed of rectilinear motion.
Indeed, since school days everyone knows that speed is a particular path x=f(t) and time t . average speed for a certain period of time:
To find out the speed of movement at a moment in time t0 you need to calculate the limit:
Rule one: set a constant
The constant can be taken out of the derivative sign. Moreover, this must be done. When solving examples in mathematics, take it as a rule - If you can simplify an expression, be sure to simplify it .
Example. Let's calculate the derivative:
Rule two: derivative of the sum of functions
The derivative of the sum of two functions is equal to the sum of the derivatives of these functions. The same is true for the derivative of the difference of functions.
We will not give a proof of this theorem, but rather consider a practical example.
Find the derivative of the function:
Rule three: derivative of the product of functions
The derivative of the product of two differentiable functions is calculated by the formula:
Example: find the derivative of a function:
Solution: 
It is important to talk about calculating derivatives of complex functions here. Derivative complex function is equal to the product of the derivative of this function with respect to the intermediate argument and the derivative of the intermediate argument with respect to the independent variable.
In the above example we come across the expression:
In this case, the intermediate argument is 8x to the fifth power. In order to calculate the derivative of such an expression, we first calculate the derivative of the external function with respect to the intermediate argument, and then multiply by the derivative of the intermediate argument itself with respect to the independent variable.
Rule four: derivative of the quotient of two functions
Formula for determining the derivative of the quotient of two functions:
We tried to talk about derivatives for dummies from scratch. This topic is not as simple as it seems, so be warned: there are often pitfalls in the examples, so be careful when calculating derivatives.
With any questions on this and other topics, you can contact the student service. Behind short term We will help you solve the most difficult tests and solve problems, even if you have never done derivative calculations before.
In this lesson we will get acquainted with the concept of a function of two variables, and also consider in detail the most common task - finding partial derivatives first and second order, complete differential of a function.
To effectively study the material below, you necessary be able to more or less confidently find “ordinary” derivatives of functions of one variable. You can learn how to handle derivatives correctly in lessons How to find the derivative? and Derivative of a complex function. We also need a table of derivatives elementary functions and the rules of differentiation, it is most convenient if it is at hand in printed form.
Let's start with the very concept of a function of two variables, we will try to limit ourselves to the minimum of theory, since the site has a practical orientation. A function of two variables is usually written as , with the variables being called independent variables or arguments.
Example: - function of two variables.
Sometimes the notation is used. There are also tasks where the letter is used instead of a letter.
It is useful to know the geometric meaning of functions. A function of one variable corresponds to a certain line on a plane, for example, the familiar school parabola. Any function of two variables with geometric point view represents a surface in three-dimensional space (planes, cylinders, balls, paraboloids, etc.). But, in fact, this is already analytical geometry, and mathematical analysis is on our agenda.
Let's move on to the question of finding partial derivatives of the first and second orders. Must report good news for those who have drunk a few cups of coffee and tuned in to unimaginably difficult material: partial derivatives are almost the same as “ordinary” derivatives of a function of one variable.
For partial derivatives, all differentiation rules and the table of derivatives of elementary functions are valid. There are just a couple of small differences, which we'll get to in a moment.
Example 1
Find the first and second order partial derivatives of the function
First, let's find the first-order partial derivatives. There are two of them.
Designations:
Or – partial derivative with respect to “x”
Or – partial derivative with respect to “y”
Let's start with .
Important! When we find the partial derivative with respect to “x”, then the variable is considered a constant (constant number).
Let's decide. In this lesson we will immediately provide the complete solution, and provide comments below.
Comments on the actions performed:
(1) The first thing we do when finding the partial derivative is to conclude all function in brackets under the prime with subscript.
Attention, important! WE DO NOT LOSE subscripts during the solution process. In this case, if you draw a “stroke” somewhere without , then the teacher, at a minimum, can put it next to the task (immediately bite off part of the point for inattention).
(2) We use the rules of differentiation 
; . For simple example like this one, both rules can easily be applied in one step. Pay attention to the first term: since is considered a constant, and any constant can be taken out of the derivative sign, then we put it out of brackets. That is, in this situation it is no better than an ordinary number. Now let's look at the third term: here, on the contrary, there is nothing to take out. Since it is a constant, it is also a constant, and in this sense it is no better than the last term - “seven”.
(2) We use the table of derivatives of elementary functions. Let’s mentally change all the “X’s” in the table to “I’s”. That is, this table is equally valid for (and for any letter in general). In this case, the formulas we use are: and .
So, the first order partial derivatives are found
The calculator calculates the derivatives of all elementary functions, giving detailed solution. The differentiation variable is determined automatically.
Derivative of a function- one of the most important concepts in mathematical analysis. The emergence of the derivative was led to such problems as, for example, calculating the instantaneous speed of a point at a moment in time, if the path depending on time is known, the problem of finding the tangent to a function at a point.
Most often, the derivative of a function is defined as the limit of the ratio of the increment of the function to the increment of the argument, if it exists.
Definition. Let the function be defined in some neighborhood of the point. Then the derivative of the function at a point is called the limit, if it exists
How to calculate the derivative of a function?
In order to learn to differentiate functions, you need to learn and understand differentiation rules and learn to use table of derivatives.
Rules of differentiation
Let and be arbitrary differentiable functions of a real variable and be some real constant. Then
— rule for differentiating the product of functions
— rule for differentiation of quotient functions
0" height="33" width="370" style="vertical-align: -12px;"> — differentiation of a function with a variable exponent
— rule for differentiating a complex function
— rule for differentiating a power function
Derivative of a function online
Our calculator will quickly and accurately calculate the derivative of any function online. The program will not make mistakes when calculating the derivative and will help you avoid long and tedious calculations. Online calculator It will also be useful in the case when there is a need to check the correctness of your solution, and if it is incorrect, quickly find the error.
Each partial derivative (by x and by y) of a function of two variables is the ordinary derivative of a function of one variable for a fixed value of the other variable:
(Where y= const),
(Where x= const).
Therefore, partial derivatives are calculated using formulas and rules for calculating derivatives of functions of one variable, while considering the other variable constant.
If you do not need an analysis of examples and the minimum theory required for this, but only need a solution to your problem, then go to online partial derivative calculator .
If it’s hard to concentrate to keep track of where the constant is in the function, then in the draft solution of the example, instead of a variable with a fixed value, you can substitute any number - then you can quickly calculate the partial derivative as the ordinary derivative of a function of one variable. You just need to remember to return the constant (a variable with a fixed value) to its place when finishing the final design.
The property of partial derivatives described above follows from the definition of a partial derivative, which may appear in exam questions. Therefore, to familiarize yourself with the definition below, you can open the theoretical reference.
Concept of continuity of function z= f(x, y) at a point is defined similarly to this concept for a function of one variable.
Function z = f(x, y) is called continuous at a point if
Difference (2) is called the total increment of the function z(it is obtained as a result of increments of both arguments).
Let the function be given z= f(x, y) and period
If the function change z occurs when only one of the arguments changes, for example, x, with a fixed value of another argument y, then the function will receive an increment
called partial increment of function f(x, y) By x.
Considering a function change z depending on changing only one of the arguments, we effectively change to a function of one variable.
If there is a finite limit
then it is called the partial derivative of the function f(x, y) by argument x and is indicated by one of the symbols
(4)
The partial increment is determined similarly z By y:
and partial derivative f(x, y) By y:
(6)
Example 1.
Solution. We find the partial derivative with respect to the variable "x":
(y fixed);
We find the partial derivative with respect to the variable "y":
(x fixed).
As you can see, it does not matter to what extent the variable is fixed: in this case it is simply a certain number that is a factor (as in the case of the ordinary derivative) of the variable with which we find the partial derivative. If the fixed variable is not multiplied by the variable with which we find the partial derivative, then this lonely constant, no matter to what extent, as in the case of the ordinary derivative, vanishes.
Example 2. Given a function
Find partial derivatives
(by X) and (by Y) and calculate their values at the point A (1; 2).
Solution. At fixed y the derivative of the first term is found as the derivative of the power function ( table of derivative functions of one variable):
.
At fixed x the derivative of the first term is found as the derivative exponential function, and the second – as a derivative of a constant:
Now let's calculate the values of these partial derivatives at the point A (1; 2):
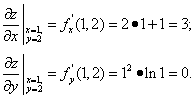
You can check the solution to partial derivative problems at online partial derivative calculator .
Example 3. Find partial derivatives of a function
Solution. In one step we find
(y x, as if the argument of sine were 5 x: in the same way, 5 appears before the function sign);
(x is fixed and is in this case a multiplier at y).
You can check the solution to partial derivative problems at online partial derivative calculator .
The partial derivatives of a function of three or more variables are defined similarly.
If each set of values ( x; y; ...; t) independent variables from the set D corresponds to one specific value u from many E, That u called a function of variables x, y, ..., t and denote u= f(x, y, ..., t).
For functions of three or more variables, there is no geometric interpretation.
Partial derivatives of a function of several variables are also determined and calculated under the assumption that only one of the independent variables changes, while the others are fixed.
Example 4. Find partial derivatives of a function
.
Solution. y And z fixed:
x And z fixed:
x And y fixed:
Find partial derivatives yourself and then look at the solutions
Example 5.
Example 6. Find partial derivatives of a function.
The partial derivative of a function of several variables has the same mechanical meaning is the same as the derivative of a function of one variable, is the rate of change of the function relative to a change in one of the arguments.
Example 8. Quantitative value of flow P passengers railways can be expressed by a function
Where P– number of passengers, N– number of residents of correspondent points, R– distance between points.
Partial derivative of a function P By R, equal
shows that the decrease in passenger flow is inversely proportional to the square of the distance between corresponding points with the same number of residents in points.
Partial derivative P By N, equal
shows that the increase in passenger flow is proportional to twice the number of residents of settlements at the same distance between points.
You can check the solution to partial derivative problems at online partial derivative calculator .
Full differential
The product of a partial derivative and the increment of the corresponding independent variable is called a partial differential. Partial differentials are denoted as follows:
The sum of partial differentials for all independent variables gives the total differential. For a function of two independent variables, the total differential is expressed by the equality
(7)
Example 9. Find the complete differential of a function
Solution. The result of using formula (7):
A function that has a total differential at every point of a certain domain is said to be differentiable in that domain.
Find the total differential yourself and then look at the solution
Just as in the case of a function of one variable, the differentiability of a function in a certain domain implies its continuity in this domain, but not vice versa.
Let us formulate without proof a sufficient condition for the differentiability of a function.
Theorem. If the function z= f(x, y) has continuous partial derivatives
in a given region, then it is differentiable in this region and its differential is expressed by formula (7).
It can be shown that, just as in the case of a function of one variable, the differential of the function is the main linear part of the increment of the function, so in the case of a function of several variables, the total differential is the main, linear with respect to the increments of independent variables, part of the total increment of the function.
For a function of two variables, the total increment of the function has the form
(8)
where α and β are infinitesimal at and .
Higher order partial derivatives
Partial derivatives and functions f(x, y) themselves are some functions of the same variables and, in turn, can have derivatives with respect to different variables, which are called partial derivatives of higher orders.
Functions of two variables, partial derivatives, differentials and gradient
Topic 5.Functions of two variables.
partial derivatives
Definition of a function of two variables, methods of setting.
Partial derivatives.
Gradient of a function of one variable
Finding the largest and smallest values of a function of two variables in a closed bounded domain
1. Definition of a function of several variables, methods of setting
For functions of two variables
domain of definition
is some set of points on a plane
, and the range of values is the interval on the axis 
.
For visual representation functions of two changes nyh are applied level lines.
Example
.
For function 
build a graph and level lines. Write down the equation of the level line passing through the point 
.
Schedule linear function is plane in space.
For a function, the graph is a plane passing through the points 
, 
, 
.
Function level lines are parallel lines whose equation is 
.
For linear function of two variables
level lines are given by the equation 
and represent a family of parallel lines on a plane.
4
Graph of a function 0 1 2 X
Function level lines
Private projectsderived functions of two variables
Consider the function 
. Let's give the variable 
at the point 
arbitrary increment 
, leaving variable value 
unchanged. Corresponding Function Increment
called private increment of a function by variable at the point 
.
Defined similarly partial function incrementby variable: .
Designationpartial derivative with respect to: 
, 
,
, 
.
Partial derivative of a function with respect to a variable 
called the final limit :
Designations: 
, 
,
, 
.
To find the partial derivative 
by variable, the rules for differentiating a function of one variable are used, assuming the variable is constant..
Similarly, to find the partial derivative with respect to a variable a variable is considered constant 
.
Example
. For function 
find partial derivatives 
, 
and calculate their values at the point 
.
Partial derivative of a function 
by variable is under the assumption that it is constant:
Let us find the partial derivative of the function with respect to , assuming constant:
Let us calculate the values of partial derivatives at 
, 
:
; 
.
Second order partial derivatives functions of several variables are called partial derivatives of first order partial derivatives.
Let us write down the 2nd order partial derivatives for the function:
; 
;
; 
.
; 
etc.
If mixed partial derivatives of functions of several variables are continuous at some point 
, then they equal to each other at this point. This means that for a function of two variables, the values of mixed partial derivatives do not depend on the order of differentiation:
.
Example.
For the function, find the second order partial derivatives 
And 
.
Solution
The mixed partial derivative is found by successively differentiating first the function 
by (assuming constant), then differentiating the derivative 
by (considering constant).
The derivative is found by first differentiating the function with respect to , then the derivative with respect to .
Mixed partial derivatives are equal to each other: 
.
3. Gradient of a function of two variables
Gradient properties
Example
. Given a function 
. Find the gradient 
at the point 
and build it.
Solution
Let's find the coordinates of the gradient - partial derivatives.
At the point 
gradient
equal to . Beginning of the vector 
at point , and the end at point .
5 
4. Finding the largest and smallest values of a function of two variables in a closed limited area
Formulation of the problem.
Let there be a closed bounded region on the plane 
is given by a system of inequalities of the form 
. It is required to find points in the region at which the function takes the largest and smallest values.
Important is problem of finding an extremum, mathematical model which contains linear restrictions (equations, inequalities) and linear function 
.
Formulation of the problem.
Find the largest and smallest values of a function 
(2.1)
under restrictions
(2.2)
. (2.3)
Since for a linear function there are not many variables critical points inside region 
, then the optimal solution, which delivers an extremum to the objective function, is achieved only on the border of the region. For a region defined by linear constraints, the points of possible extremum are corner points. This allows us to consider the solution to the problem graphical method.
Graphical solution of a system of linear inequalities
For graphic solution For this problem, you must be able to solve graphically systems of linear inequalities with two variables.
Procedure:
Note that the inequality 
defines right coordinate half-plane(from axis 
), and the inequality 
- upper coordinate half-plane(from axis 
).
Example.
Solve graphically the inequality 
.
Let us write down the equation of the boundary line 
and build it based on two points, for example, 
And 
. A straight line divides a plane into two half-planes.
Point coordinates 
satisfy the inequality ( 
– true), which means that the coordinates of all points of the half-plane containing the point satisfy the inequality. The solution to the inequality will be the coordinates of the points of the half-plane located to the right of the boundary line, including points on the boundary. The desired half-plane is highlighted in the figure.
Solution 
system of inequalities is called acceptable, if its coordinates are non-negative, . The set of feasible solutions to the system of inequalities forms a region that is located in the first quarter of the coordinate plane.
Example. Construct the solution domain of the system of inequalities
The solutions to the inequalities are:
1) 
- half-plane located to the left and below relative to the straight line ( 
) 
;
2) 
– half-plane located in the lower-right half-plane relative to the straight line ( 
) 
;
3) 
- half-plane located to the right of the straight line ( 
) 
;
4) - half-plane above the x-axis, that is, straight line ( 
) 
.
0 
Range of feasible solutions of a given system of linear inequalities is a set of points located inside and on the boundary of the quadrilateral 
, which is intersection four half-planes.
Geometric representation of a linear function
(level lines and gradient)
Let's fix the value 
, we get the equation 
, which geometrically defines a straight line. At each point on the line the function takes the value 
and is level line. Giving 
different meanings, For example, 
, ... , we get a lot of level lines - set of parallel
direct.
Let's build gradient- vector 
, whose coordinates are equal to the values of the coefficients of the variables in the function 
. This vector: 1) perpendicular to each straight line (level line) 
; 2) shows the direction of increase of the objective function.
Example
. Plot level lines and gradient functions 
.
Level lines at , , are straight 
, 
, 
, parallel to each other. The gradient is a vector perpendicular to each level line.
Graphically finding the largest and smallest values of a linear function in an area
Geometric formulation of the problem. Find in the solution domain of the system of linear inequalities the point through which the level line passes, corresponding to the largest (smallest) value of a linear function with two variables.
Sequencing:
4. Find the coordinates of point A by solving the system of equations of lines intersecting at point A, and calculate smallest value functions 
. Likewise for point B and highest value functions 
. built on points.variables Privatederivativesfunctions several variables and differentiation technique. Extremum functionstwovariables and its necessary...